对称加密算法是应用较早的加密算法,数据发送方将明文和密钥经加密算法处理,使其变成密文发送出去;接收方收到密文后,使用和加密算法相同的密钥进行逆算法解密,还原出明文。在对称加密算法中,使用的密钥只有一个,收发双方使用相同的密钥对数据进行加密或解密。
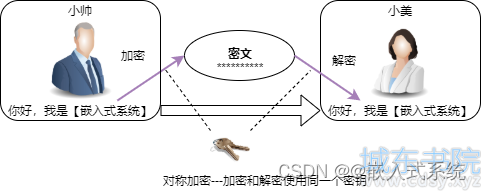
双方都必须保管好密钥,任一方的密钥泄露,都会导致加密信息不安全;尤其是双方协商更换密钥过程中,密钥会出现在传输过程中,严重影响数据的安全性。
和对称加密算法最大的区别是,非对称加密算法需要两个密钥,公开密钥(public key 简称公钥)和私有密钥(private key 简称私钥),且公钥与私钥是互相关联的一对。使用公钥对数据进行加密,只有用对应的私钥才能解密,私钥加密签名也只有公钥能解密验签。
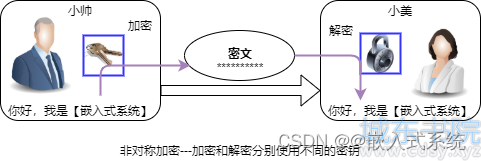
非对称加密算法实现机密信息交换的基本过程:
1、甲方生成一对密钥并将公钥公开,私钥保密
2、乙方使用甲方的提供的公钥,对机密信息加密后再发送给甲方;甲方使用自己私钥对加密后的信息进行解密
3、甲方也可以使用自己的私钥对机密信息进行签名后再发送给乙方,乙方用甲方的提供公钥对甲方发来的密文进行验签
非对称加密算法的特点:
1、公钥公开,私钥私藏,无需双方传输密钥协商,所以安全性比对称加密算法更高
2、非对称加密的算法复杂,运算速度比对称加密解密的速度慢很多
3、一般情况下使用非对称加密保护对称加密的密钥,密钥协商后使用对称加密进行通信
非对称加密算法中最常用的当属 RSA ,其算法本身基于一个简单的数论知识,给出两个素数,很容易将它们相乘,然而给出它们的乘积,想得到这两个素数就显得尤为困难。具体的私钥与公钥生成原理和加密、解密过程,不是本文关注的重点。
私钥和公钥的生成,可以借助mbedtls源码或openSSL工具生成,举例如下:
1、安装openSSL,下载地址
https://www.openssl.org/
2、安装后进入openSSL命令行界面,生成RSA2048的私钥,存入private.key文件
OpenSSL>genrsa -out private.key 2048
3、基于公钥生成私钥,存入文件public.key
OpenSSL> rsa -in private.key -pubout -out public.key
4、有些算法库采用传入指数、模数方式进行加解密,而前面生成的公私钥是PEM格式,需要变成Exponent、Modulus形式,就可以使用以下工具在线转换。
https://www.oren.net.cn/rsa/info.html
以下是RSA2048的C源码和验证范例,基于Qt测试,也可以结合硬件性能改为RSA1024,移植时注意适配形如 portable_***的三个API。
- /************************************/
- //关注微信公众号 嵌入式系统
- /************************************/
- //rsa.h
- #include "stdlib.h"
-
- #define RSA_ENCODE_LEN (2048/8) //RSA2048即256字节,可以视硬件情况改为1024
-
- typedef unsigned char uint8_t;
- typedef unsigned short int uint16_t;
- typedef unsigned int uint32_t;
-
- #define BI_MAXLEN 130
- #define DEC 10
- #define HEX 16
-
- #define CARRYOVER 0x10000
- #define CARRYLAST 0xFFFF
-
- typedef struct
- {
- uint32_t m_nLength; //大数在0x1 00 00 00 00进制下的长度
- uint16_t m_ulValue[BI_MAXLEN]; //用数组记录大数在0x100000000进制下每一位的值
- } CBigInt;
-
-
- //rsa.c
- #include "rsa.h"
- #include "time.h"
-
- /******************* 适配API *******************/
- #define portable_malloc malloc
- #define portable_free free
-
- //随机数种子源
- uint32_t portable_rand_seed(void)
- {
- time_t timestamp;
- time(×tamp);
- return timestamp;
- }
- /******************* 适配API *******************/
-
- /*****************************************************************
- 基本操作与运算
- Init, 构造大数对象并初始化为零
- Mov,赋值运算,可赋值为大数或普通整数,可重载为运算符“=”
- Cmp,比较运算,可重载为运算符“==”、“!=”、“>=”、“<=”等
- Add,加,求大数与大数或大数与普通整数的和,可重载为运算符“+”
- Sub,减,求大数与大数或大数与普通整数的差,可重载为运算符“-”
- Mul,乘,求大数与大数或大数与普通整数的积,可重载为运算符“*”
- Div,除,求大数与大数或大数与普通整数的商,可重载为运算符“/”
- Mod,模,求大数与大数或大数与普通整数的模,可重载为运算符“%”
- *****************************************************************/
- static CBigInt *Mov_Big_Long(CBigInt *X, uint32_t A);
- static CBigInt *Mov_Big_Big(CBigInt *X, CBigInt *A);
- static CBigInt *Add_Big_Big(CBigInt *X, CBigInt *A);
- static CBigInt *Sub_Big_Big(CBigInt *X, CBigInt *A);
- static CBigInt *Mul_Big_Big(CBigInt *X, CBigInt *A);
- static CBigInt *Div_Big_Big(CBigInt *X, CBigInt *A);
- static CBigInt *Mod_Big_Big(CBigInt *X, CBigInt *A);
- static CBigInt *Add_Big_Long(CBigInt *X, uint32_t A);
- static CBigInt *Sub_Big_Long(CBigInt *X, uint32_t A);
- static CBigInt *Mul_Big_Long(CBigInt *X, uint32_t A);
- static CBigInt *Div_Big_Long(CBigInt *X, uint32_t A);
- static uint32_t Mod_Big_Long(CBigInt *N, uint32_t A);
- static int Cmp(CBigInt *N, CBigInt *A);
-
- /*****************************************************************
- 输入输出
- Get,从字符串按10进制或16进制格式输入到大数
- Put,将大数按10进制或16进制格式输出到字符串
- *****************************************************************/
- static CBigInt *Get(CBigInt *N, char *str, uint32_t system);
- static char *Put(CBigInt *N, uint32_t system);
-
- /*****************************************************************
- RSA相关运算
- Rab,拉宾米勒算法进行素数测试
- Euc,欧几里德算法求解同余方程
- RsaTrans,反复平方算法进行幂模运算
- GetPrime,产生指定长度的随机大素数
- *****************************************************************/
- static int Rab(CBigInt *N);
- static CBigInt *Euc(CBigInt *X, CBigInt *A);
- static CBigInt *RsaTrans(CBigInt *X, CBigInt *A, CBigInt *B);
- static CBigInt *GetPrime(CBigInt *X, int bits);
-
-
- /*****************************************************************
- 大数运算库源文件:BigInt.c
- 说明:适用于C,linux系统 1024位RSA运算
- *****************************************************************/
- //小素数表
- const static int PrimeTable[550] =
- {
- 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73,
- 79, 83, 89, 97, 101, 103, 107, 109, 113, 127, 131, 137, 139, 149, 151, 157, 163, 167, 173, 179,
- 181, 191, 193, 197, 199, 211, 223, 227, 229, 233, 239, 241, 251, 257, 263, 269, 271, 277, 281, 283,
- 293, 307, 311, 313, 317, 331, 337, 347, 349, 353, 359, 367, 373, 379, 383, 389, 397, 401, 409, 419,
- 421, 431, 433, 439, 443, 449, 457, 461, 463, 467, 479, 487, 491, 499, 503, 509, 521, 523, 541, 547,
- 557, 563, 569, 571, 577, 587, 593, 599, 601, 607, 613, 617, 619, 631, 641, 643, 647, 653, 659, 661,
- 673, 677, 683, 691, 701, 709, 719, 727, 733, 739, 743, 751, 757, 761, 769, 773, 787, 797, 809, 811,
- 821, 823, 827, 829, 839, 853, 857, 859, 863, 877, 881, 883, 887, 907, 911, 919, 929, 937, 941, 947,
- 953, 967, 971, 977, 983, 991, 997, 1009, 1013, 1019, 1021, 1031, 1033, 1039, 1049, 1051, 1061, 1063, 1069, 1087,
- 1091, 1093, 1097, 1103, 1109, 1117, 1123, 1129, 1151, 1153, 1163, 1171, 1181, 1187, 1193, 1201, 1213, 1217, 1223, 1229,
- 1231, 1237, 1249, 1259, 1277, 1279, 1283, 1289, 1291, 1297, 1301, 1303, 1307, 1319, 1321, 1327, 1361, 1367, 1373, 1381,
- 1399, 1409, 1423, 1427, 1429, 1433, 1439, 1447, 1451, 1453, 1459, 1471, 1481, 1483, 1487, 1489, 1493, 1499, 1511, 1523,
- 1531, 1543, 1549, 1553, 1559, 1567, 1571, 1579, 1583, 1597, 1601, 1607, 1609, 1613, 1619, 1621, 1627, 1637, 1657, 1663,
- 1667, 1669, 1693, 1697, 1699, 1709, 1721, 1723, 1733, 1741, 1747, 1753, 1759, 1777, 1783, 1787, 1789, 1801, 1811, 1823,
- 1831, 1847, 1861, 1867, 1871, 1873, 1877, 1879, 1889, 1901, 1907, 1913, 1931, 1933, 1949, 1951, 1973, 1979, 1987, 1993,
- 1997, 1999, 2003, 2011, 2017, 2027, 2029, 2039, 2053, 2063, 2069, 2081, 2083, 2087, 2089, 2099, 2111, 2113, 2129, 2131,
- 2137, 2141, 2143, 2153, 2161, 2179, 2203, 2207, 2213, 2221, 2237, 2239, 2243, 2251, 2267, 2269, 2273, 2281, 2287, 2293,
- 2297, 2309, 2311, 2333, 2339, 2341, 2347, 2351, 2357, 2371, 2377, 2381, 2383, 2389, 2393, 2399, 2411, 2417, 2423, 2437,
- 2441, 2447, 2459, 2467, 2473, 2477, 2503, 2521, 2531, 2539, 2543, 2549, 2551, 2557, 2579, 2591, 2593, 2609, 2617, 2621,
- 2633, 2647, 2657, 2659, 2663, 2671, 2677, 2683, 2687, 2689, 2693, 2699, 2707, 2711, 2713, 2719, 2729, 2731, 2741, 2749,
- 2753, 2767, 2777, 2789, 2791, 2797, 2801, 2803, 2819, 2833, 2837, 2843, 2851, 2857, 2861, 2879, 2887, 2897, 2903, 2909,
- 2917, 2927, 2939, 2953, 2957, 2963, 2969, 2971, 2999, 3001, 3011, 3019, 3023, 3037, 3041, 3049, 3061, 3067, 3079, 3083,
- 3089, 3109, 3119, 3121, 3137, 3163, 3167, 3169, 3181, 3187, 3191, 3203, 3209, 3217, 3221, 3229, 3251, 3253, 3257, 3259,
- 3271, 3299, 3301, 3307, 3313, 3319, 3323, 3329, 3331, 3343, 3347, 3359, 3361, 3371, 3373, 3389, 3391, 3407, 3413, 3433,
- 3449, 3457, 3461, 3463, 3467, 3469, 3491, 3499, 3511, 3517, 3527, 3529, 3533, 3539, 3541, 3547, 3557, 3559, 3571, 3581,
- 3583, 3593, 3607, 3613, 3617, 3623, 3631, 3637, 3643, 3659, 3671, 3673, 3677, 3691, 3697, 3701, 3709, 3719, 3727, 3733,
- 3739, 3761, 3767, 3769, 3779, 3793, 3797, 3803, 3821, 3823, 3833, 3847, 3851, 3853, 3863, 3877, 3881, 3889, 3907, 3911,
- 3917, 3919, 3923, 3929, 3931, 3943, 3947, 3967, 3989, 4001
- };
-
- /****************************************************************************************
- 大数比较
- 调用方式:Cmp(N,A)
- 返回值:若N<A返回-1;若N=A返回0;若N>A返回1
- ****************************************************************************************/
- static int Cmp(CBigInt *N, CBigInt *A)
- {
- int i;
- if(N->m_nLength > A->m_nLength)
- {
- return 1;
- }
- if(N->m_nLength < A->m_nLength)
- {
- return -1;
- }
- for(i = N->m_nLength - 1; i >= 0; i--)
- {
- if(N->m_ulValue[i] > A->m_ulValue[i])
- {
- return 1;
- }
- if(N->m_ulValue[i] < A->m_ulValue[i])
- {
- return -1;
- }
- }
- return 0;
- }
-
- /****************************************************************************************
- 大数赋值
- 调用方式:__Mov_Big_Big(A)
- 返回值:N,被赋值为A
- ****************************************************************************************/
- static CBigInt *Mov_Big_Big(CBigInt *X, CBigInt *A)
- {
- memcpy(X, A, sizeof(CBigInt));
- return X;
- }
-
- static CBigInt *Mov_Big_Long(CBigInt *N, uint32_t A)
- {
- int i;
- if(A > CARRYLAST)
- {
- N->m_nLength = 2;
- N->m_ulValue[1] = (uint16_t)(A >> 16);
- N->m_ulValue[0] = (uint16_t)A;
- }
- else
- {
- N->m_nLength = 1;
- N->m_ulValue[0] = (uint16_t)A;
- }
- memset((unsigned char*)&N->m_ulValue[N->m_nLength], 0, sizeof(uint16_t) * (BI_MAXLEN - N->m_nLength));
- return N;
- }
-
- /****************************************************************************************
- 大数相加
- 调用形式:Add_Big_Big(X,A)
- 返回值:X=X+A
- ****************************************************************************************/
- static CBigInt *Add_Big_Big(CBigInt *X, CBigInt *A)
- {
- uint32_t i;
- uint16_t carry = 0;
- uint32_t sum = 0;
- if(X->m_nLength < A->m_nLength)
- {
- X->m_nLength = A->m_nLength;
- }
- for(i = 0; i < X->m_nLength; i++)
- {
- sum = A->m_ulValue[i];
- sum = sum + X->m_ulValue[i] + carry;
- X->m_ulValue[i] = (uint16_t)sum;
- carry = (uint16_t)(sum >> 16);
- }
- X->m_ulValue[X->m_nLength] = carry;
- X->m_nLength += carry;
- return X;
- }
-
- static CBigInt *Add_Big_Long(CBigInt *X, uint32_t A)
- {
- uint32_t sum;
- sum = X->m_ulValue[0];
- sum += A;
- X->m_ulValue[0] = (uint16_t)sum;
- if(sum > CARRYLAST)
- {
- uint32_t i = 1;
- while(X->m_ulValue[i] == CARRYLAST)
- {
- X->m_ulValue[i] = 0;
- i++;
- }
- X->m_ulValue[i]++;
- if(X->m_nLength == i)
- {
- X->m_nLength++;
- }
- }
- return X;
- }
-
- /****************************************************************************************
- 大数相减
- 调用形式:Sub_Big_Big(X,A)
- 返回值:X=X-A
- ****************************************************************************************/
- static CBigInt *Sub_Big_Big(CBigInt *X, CBigInt *A)
- {
- if(Cmp(X, A) <= 0)
- {
- memset(X, 0, sizeof(CBigInt));
- return X;
- }
- else
- {
- uint16_t carry = 0;
- uint32_t num;
- uint32_t i;
- for(i = 0; i < X->m_nLength; i++)
- {
- if((X->m_ulValue[i] > A->m_ulValue[i]) || ((X->m_ulValue[i] == A->m_ulValue[i]) && (carry == 0)))
- {
- X->m_ulValue[i] = X->m_ulValue[i] - carry - A->m_ulValue[i];
- carry = 0;
- }
- else
- {
- num = CARRYOVER + X->m_ulValue[i];
- X->m_ulValue[i] = (uint32_t)(num - carry - A->m_ulValue[i]);
- carry = 1;
- }
- }
- while(X->m_ulValue[X->m_nLength - 1] == 0)
- {
- X->m_nLength--;
- }
- return X;
- }
- }
-
- static CBigInt *Sub_Big_Long(CBigInt *X, uint32_t A)
- {
- if(X->m_ulValue[0] >= A)
- {
- X->m_ulValue[0] -= A;
- return X;
- }
- if(X->m_nLength == 1)
- {
- memset(X, 0, sizeof(CBigInt));
- return X;
- }
- else
- {
- uint32_t num = CARRYOVER + X->m_ulValue[0];
- int i = 1;
- X->m_ulValue[0] = (uint16_t)(num - A);
- while(X->m_ulValue[i] == 0)
- {
- X->m_ulValue[i] = CARRYLAST;
- i++;
- }
- X->m_ulValue[i]--;
- if(X->m_ulValue[i] == 0)
- {
- X->m_nLength--;
- }
- return X;
- }
- }
-
- /****************************************************************************************
- 大数相乘
- 调用形式:Mul_Big_Big(N,A)
- 返回值:X=N*A
- A a 0
- N c d
- 0 d*0
- 1 c*0
- d*a
- 2 c*a
-
- ****************************************************************************************/
- static CBigInt *Mul_Big_Big(CBigInt *X, CBigInt *A)
- {
- if(A->m_nLength == 1)
- {
- return Mul_Big_Long(X, A->m_ulValue[0]);
- }
- else
- {
- uint32_t sum, mul = 0, carry = 0;
- uint32_t i, j;
- CBigInt N = {0};
- memcpy(&N, X, sizeof(CBigInt));
- memset(X, 0, sizeof(CBigInt));
- X->m_nLength = N.m_nLength + A->m_nLength - 1;
- for(i = 0; i < X->m_nLength; i++)
- {
- sum = carry;
- carry = 0;
- for(j = 0; j < A->m_nLength; j++)
- {
- if(((i - j) >= 0) && ((i - j) < N.m_nLength))
- {
- mul = N.m_ulValue[i - j];
- mul *= A->m_ulValue[j];
- carry += mul >> 16;
- mul = mul & CARRYLAST;
- sum += mul;
- }
- }
- carry += sum >> 16;
- X->m_ulValue[i] = (uint16_t)sum;
- }
- if(carry)
- {
- X->m_nLength++;
- X->m_ulValue[X->m_nLength - 1] = (uint16_t)carry;
- }
- return X;
- }
- }
-
- static CBigInt *Mul_Big_Long(CBigInt *X, uint32_t A)
- {
- uint32_t mul;
- uint32_t carry = 0;
- uint32_t i;
- for(i = 0; i < X->m_nLength; i++)
- {
- mul = X->m_ulValue[i];
- mul = mul * A + carry;
- X->m_ulValue[i] = (uint16_t)mul;
- carry = (uint16_t)(mul >> 16);
- }
- if(carry)
- {
- X->m_nLength++;
- X->m_ulValue[X->m_nLength - 1] = carry;
- }
- return X;
- }
-
- /****************************************************************************************
- 大数相除
- 调用形式:Div_Big_Big(N,A)
- 返回值:X=N/A
- ****************************************************************************************/
- static CBigInt *Div_Big_Big(CBigInt *X, CBigInt *A)
- {
- CBigInt Y = {0}, Z = {0}, T;
- if(A->m_nLength == 1)
- {
- return Div_Big_Long(X, A->m_ulValue[0]);
- }
- else
- {
- uint32_t i, len;
- uint32_t num, div;
- memcpy(&Y, X, sizeof(CBigInt));
- while(Cmp(&Y, A) >= 0)
- {
- div = Y.m_ulValue[Y.m_nLength - 1];
- num = A->m_ulValue[A->m_nLength - 1];
- len = Y.m_nLength - A->m_nLength;
- if((div == num) && (len == 0))
- {
- Add_Big_Long(X, 1);
- break;
- }
- if((div <= num) && len)
- {
- len--;
- div = (div << 16) + Y.m_ulValue[Y.m_nLength - 2];
- }
- div = div / (num + 1);
- Mov_Big_Long(&Z, div);
- if(len)
- {
- Z.m_nLength += len;
- for(i = Z.m_nLength - 1; i >= len; i--)
- {
- Z.m_ulValue[i] = Z.m_ulValue[i - len];
- }
- for(i = 0; i < len; i++)
- {
- Z.m_ulValue[i] = 0;
- }
- }
- Add_Big_Big(X, &Z);
- memcpy(&T, A, sizeof(CBigInt));
- Mul_Big_Big(&T, &Z);
- Sub_Big_Big(&Y, &T);
- }
- return X;
- }
- }
- static CBigInt *Div_Big_Long(CBigInt *X, uint32_t A)
- {
- if(X->m_nLength == 1)
- {
- X->m_ulValue[0] = X->m_ulValue[0] / A;
- return X;
- }
- else
- {
- uint32_t div, mul;
- uint32_t carry = 0;
- int i;
- for(i = X->m_nLength - 1; i >= 0; i--)
- {
- div = carry;
- div = (div << 16) + X->m_ulValue[i];
- X->m_ulValue[i] = (uint16_t)(div / A);
- mul = (div / A) * A;
- carry = (uint16_t)(div - mul);
- }
- if(X->m_ulValue[X->m_nLength - 1] == 0)
- {
- X->m_nLength--;
- }
- return X;
- }
- }
-
- /****************************************************************************************
- 大数求模
- 调用形式:Mod_Big_Big(N,A)
- 返回值:X=N%A
- ****************************************************************************************/
- static CBigInt *Mod_Big_Big(CBigInt *X, CBigInt *A)
- {
- CBigInt Y = {0}, Z;
- uint32_t div, num;
- uint32_t carry = 0;
- uint32_t i, len;
- while(Cmp(X, A) >= 0)
- {
- div = X->m_ulValue[X->m_nLength - 1];
- num = A->m_ulValue[A->m_nLength - 1];
- len = X->m_nLength - A->m_nLength;
- if((div == num) && (len == 0))
- {
- Sub_Big_Big(X, A);
- break;
- }
- if((div <= num) && len)
- {
- len--;
- div = (div << 16) + X->m_ulValue[X->m_nLength - 2];
- }
- div = div / (num + 1);
- Mov_Big_Long(&Y, div);
- memcpy(&Z, A, sizeof(CBigInt));
- Mul_Big_Big(&Z, &Y);
- memcpy(&Y, &Z, sizeof(CBigInt));
- if(len)
- {
- Y.m_nLength += len;
- for(i = Y.m_nLength - 1; i >= len; i--)
- {
- Y.m_ulValue[i] = Y.m_ulValue[i - len];
- }
- for(i = 0; i < len; i++)
- {
- Y.m_ulValue[i] = 0;
- }
- }
- Sub_Big_Big(X, &Y);
- }
- return X;
- }
-
- static uint32_t Mod_Big_Long(CBigInt *N, uint32_t A)
- {
- if(N->m_nLength == 1)
- {
- return(N->m_ulValue[0] % A);
- }
- else
- {
- uint32_t div;
- uint32_t carry = 0;
- int i;
- for(i = N->m_nLength - 1; i >= 0; i--)
- {
- div = N->m_ulValue[i];
- div += carry * CARRYOVER;
- carry = (uint16_t)(div % A);
- }
- return carry;
- }
- }
-
- /****************************************************************************************
- 从字符串按10进制或16进制格式输入到大数
- 调用格式:Get(N,str,sys)
- 返回值:N被赋值为相应大数
- sys暂时只能为10或16
- ****************************************************************************************/
- static CBigInt *Get(CBigInt *N, char *s, uint32_t system)
- {
- int i;
- int len = strlen(s), k;
- memset(N, 0, sizeof(CBigInt));
- N->m_nLength = 1;
- for(i = 0; i < len; i++)
- {
- Mul_Big_Long(N, system);
- if((s[i] >= '0') && (s[i] <= '9'))
- {
- k = s[i] - 48;
- }
- else if((s[i] >= 'A') && (s[i] <= 'F'))
- {
- k = s[i] - 55;
- }
- else if((s[i] >= 'a') && (s[i] <= 'f'))
- {
- k = s[i] - 87;
- }
- else
- {
- k = 0;
- }
- Add_Big_Long(N, k);
- }
- return N;
- }
- static CBigInt *GetHex(CBigInt *N, unsigned char *s, unsigned short len, uint32_t system)
- {
- int i, j;
- unsigned char *p = (unsigned char*)N->m_ulValue;
- memset(N, 0, sizeof(CBigInt));
- N->m_nLength = 1;
- for(i = len - 1, j = 0; i >= 0; i--, j++)
- {
- p[j] = s[i];
- }
- i = len % 2;
- if(i > 0)
- {
- N->m_nLength = len / 2 + 1;
- }
- else
- {
- N->m_nLength = len / 2;
- }
- return N;
- }
- /****************************************************************************************
- 将大数按10进制或16进制格式输出为字符串
- 调用格式:Put(N,str,sys)
- 返回值:无,参数str被赋值为N的sys进制字符串
- sys暂时只能为10或16
- ****************************************************************************************/
- static char *Put(CBigInt *N, uint32_t system)
- {
- char t[17] = "0123456789ABCDEF";
- int i, a;
- static char s[2048];
-
- if((N->m_nLength == 1) && (N->m_ulValue[0] == 0))
- {
- return NULL;
- }
- else
- {
- CBigInt X = {0};
- memcpy(&X, N, sizeof(CBigInt));
- memset(s, 0, 2048);
- for(i = 2046; X.m_ulValue[X.m_nLength - 1] > 0 && i > 0; i--)
- {
- a = Mod_Big_Long(&X, system);
- s[i] = t[a];
- Div_Big_Long(&X, system);
- }
- if(i % 2 == 0)
- {
- return &s[i + 1];
- }
- else
- {
- s[i] = '0';
- return &s[i];
- }
- }
- }
-
- static void PutHex(CBigInt *N, uint8_t *out, uint16_t *len)
- {
- int i, j, size;
- if((N->m_nLength == 1) && (N->m_ulValue[0] == 0))
- {
- return;
- }
- size = N->m_nLength * sizeof(N->m_ulValue[0]);
- for(i = size - 1, j = 0; i >= 0; i--, j++)
- {
- out[j] = ((uint8_t*)N->m_ulValue)[i];
- }
- *len = size;
- }
-
- /****************************************************************************************
- 求不定方程ax-by=1的最小整数解
- 调用方式:Euc(N,A)
- 返回值:X,满足:NX mod A=1
- ****************************************************************************************/
- static CBigInt *Euc(CBigInt *X, CBigInt *A)
- {
- CBigInt M = {0}, E = {0}, N = {0}, Y = {0}, I = {0}, J = {0};
- int x, y;
- memcpy(&E, X, sizeof(CBigInt));
- memcpy(&M, A, sizeof(CBigInt));
- Mov_Big_Long(X, 0);
- Mov_Big_Long(&Y, 1);
- x = y = 1;
- while((E.m_nLength != 1) || (E.m_ulValue[0] != 0))
- {
- memcpy(&I, &M, sizeof(CBigInt));
- Div_Big_Big(&I, &E);
- memcpy(&J, &M, sizeof(CBigInt));
- Mod_Big_Big(&J, &E);
- memcpy(&M, &E, sizeof(CBigInt));
- memcpy(&E, &J, sizeof(CBigInt));
- memcpy(&J, &Y, sizeof(CBigInt));
- Mul_Big_Big(&Y, &I);
- if(x == y)
- {
- if(Cmp(X, &Y) >= 0)
- {
- Sub_Big_Big(&Y, X);
- }
- else
- {
- Sub_Big_Big(&Y, X);
- y = 0;
- }
- }
- else
- {
- Add_Big_Big(&Y, X);
- x = 1 - x;
- y = 1 - y;
- }
- memcpy(X, &J, sizeof(CBigInt));
- }
- if(x == 0)
- {
- Sub_Big_Big(X, A);
- }
- return X;
- }
-
- /****************************************************************************************
- 求乘方的模
- 调用方式:RsaTrans(N,A,B)
- 返回值:X=N^A MOD B
- ****************************************************************************************/
- static CBigInt *RsaTrans(CBigInt *X, CBigInt *A, CBigInt *B)
- {
- CBigInt N = {0}, Y = {0}, Z;
- int i, j, k;
- uint32_t n;
- uint32_t num;
- k = A->m_nLength * 16 - 16;
- num = A->m_ulValue[A->m_nLength - 1];
- while(num)
- {
- num = num >> 1;
- k++;
- }
- memcpy(&N, X, sizeof(CBigInt));
- for(i = k - 2; i >= 0; i--)
- {
- memcpy(&Y, X, sizeof(CBigInt));
- Mul_Big_Long(&Y, X->m_ulValue[X->m_nLength - 1]);
- Mod_Big_Big(&Y, B);
- for(n = 1; n < X->m_nLength; n++)
- {
- for(j = Y.m_nLength; j > 0; j--)
- {
- Y.m_ulValue[j] = Y.m_ulValue[j - 1];
- }
- Y.m_ulValue[0] = 0;
- Y.m_nLength++;
- memcpy(&Z, X, sizeof(CBigInt));
- Mul_Big_Long(&Z, X->m_ulValue[X->m_nLength - n - 1]);
- Add_Big_Big(&Y, &Z);
- Mod_Big_Big(&Y, B);
- }
- memcpy(X, &Y, sizeof(CBigInt));
- if((A->m_ulValue[i >> 4] >> (i & 15)) & 1)
- {
- memcpy(&Y, &N, sizeof(CBigInt));
- Mul_Big_Long(&Y, X->m_ulValue[X->m_nLength - 1]);
- Mod_Big_Big(&Y, B);
- for(n = 1; n < X->m_nLength; n++)
- {
- for(j = Y.m_nLength; j > 0; j--)
- {
- Y.m_ulValue[j] = Y.m_ulValue[j - 1];
- }
- Y.m_ulValue[0] = 0;
- Y.m_nLength++;
- memcpy(&Z, &N, sizeof(CBigInt));
- Mul_Big_Long(&Z, X->m_ulValue[X->m_nLength - n - 1]);
- Add_Big_Big(&Y, &Z);
- Mod_Big_Big(&Y, B);
- }
- memcpy(X, &Y, sizeof(CBigInt));
- }
- }
- return X;
- }
-
- /****************************************************************************************
- 拉宾米勒算法测试素数
- 调用方式:Rab(N)
- 返回值:若N为素数,返回1,否则返回0
- ****************************************************************************************/
- static int Rab(CBigInt *N)
- {
- CBigInt S = {0}, A = {0}, I = {0}, K = {0};
- uint32_t i, j, pass;
- for(i = 0; i < 550; i++)
- {
- if(Mod_Big_Long(N, PrimeTable[i]) == 0)
- {
- return 0;
- }
- }
- memcpy(&K, N, sizeof(CBigInt));
- K.m_ulValue[0]--;
- for(i = 0; i < 5; i++)
- {
- pass = 0;
- Mov_Big_Long(&A, rand()*rand());
- memcpy(&S, &K, sizeof(CBigInt));
- while((S.m_ulValue[0] & 1) == 0)
- {
- for(j = 0; j < S.m_nLength; j++)
- {
- S.m_ulValue[j] = S.m_ulValue[j] >> 1;
- if(S.m_ulValue[j + 1] & 1)
- {
- S.m_ulValue[j] = S.m_ulValue[j] | 0x8000;
- }
- }
- if(S.m_ulValue[S.m_nLength - 1] == 0)
- {
- S.m_nLength--;
- }
- memcpy(&I, &A, sizeof(CBigInt));
- RsaTrans(&I, &S, N);
- if(Cmp(&I, &K) == 0)
- {
- pass = 1;
- break;
- }
- }
- if((I.m_nLength == 1) && (I.m_ulValue[0] == 1))
- {
- pass = 1;
- }
- if(pass == 0)
- {
- return 0;
- }
- }
- return 1;
- }
-
- /****************************************************************************************
- 产生随机素数
- 调用方法:GetPrime(N,bits)
- 返回值:N,被赋值为一个bits位(0x100000000进制长度)的素数
- ****************************************************************************************/
- static CBigInt *GetPrime(CBigInt *N, int bits)
- {
- uint32_t i;
- CBigInt S = {0}, A = {0}, I = {0}, K = {0};
-
- memset(N, 0, sizeof(CBigInt));
- N->m_nLength = bits;
- begin:
- srand(portable_rand_seed());
- for(i = 0; i < N->m_nLength; i++)
- {
- N->m_ulValue[i] = rand() * 0x100 + rand();
- }
- N->m_ulValue[0] = N->m_ulValue[0] | 1;
- for(i = N->m_nLength - 1; i > 0; i--)
- {
- N->m_ulValue[i] = N->m_ulValue[i] << 1;
- if(N->m_ulValue[i - 1] & 0x8000)
- {
- N->m_ulValue[i]++;
- }
- }
- N->m_ulValue[0] = N->m_ulValue[0] << 1;
- N->m_ulValue[0]++;
- for(i = 0; i < 550; i++)
- {
- if(Mod_Big_Long(N, PrimeTable[i]) == 0)
- {
- goto begin;
- }
- }
- memcpy(&K, N, sizeof(CBigInt));
- K.m_ulValue[0]--;
- for(i = 0; i < 5; i++)
- {
- Mov_Big_Long(&A, rand()*rand());
- memcpy(&S, &K, sizeof(CBigInt));
- Div_Big_Long(&S, 2);
- memcpy(&I, &A, sizeof(CBigInt));
- RsaTrans(&I, &S, N);
- if(((I.m_nLength != 1) || (I.m_ulValue[0] != 1)) && (Cmp(&I, &K) != 0))
- {
- goto begin;
- }
- }
- return N;
- }
-
- /***********************************************************************/
-
- static void entropy_poll(unsigned char *output, unsigned int len)
- {
- if(len > 0)
- {
- int i;
- srand(portable_rand_seed);
- for(i = 0; i < len; i++)
- {
- output[i] = rand() % 0xff + 1;
- }
- }
- }
-
- static char *del_PKCS1Padding(char *src)
- {
- int len = strlen(src);
- if(len % 2 == 1)
- {
- src++;
- }
- while(*src != 0 && *(src + 1) != 0)
- {
- if(*src == '0' && *(src + 1) == '0')
- {
- src += 2;
- break;
- }
- src += 2;
- }
- return src;
- }
-
- static int add_PKCS1Padding(unsigned char *src, unsigned int len, unsigned char *out)
- {
- if(len > RSA_ENCODE_LEN - 11)
- {
- return -1;
- }
- else
- {
- /*要加密的msg*/
- memcpy(&out[RSA_ENCODE_LEN - len], src, len);
- out[0] = 0;
- out[1] = 2;
- /*至少8字节的随机数*/
- entropy_poll(&out[2], RSA_ENCODE_LEN - 3 - len);
- out[RSA_ENCODE_LEN - len - 1] = 0;
- return 0;
- }
- }
-
- static int PKCS1PKCS1PaddingHexRemove(unsigned char *input, unsigned short *len, unsigned char *output)
- {
- if(input[0] == 0 && (input[1] == 1 || input[1] == 2))
- {
- int i;
- for(i = 2; i < *len; i++)
- {
- if(input[i] == 0)
- {
- *len -= (i + 1);
- memcpy(output, &input[i + 1], *len);
- return *len;
- }
- }
- }
- return -1;
- }
-
-
- int RSA2048_pri_PKCS1Padding_Encode(unsigned char *data, unsigned short len, unsigned char *out, char *publicKey, char *ModulusHex)
- {
- unsigned char buf[RSA_ENCODE_LEN];
- CBigInt N, E;
- CBigInt mw, mi, jm;
- uint16_t outlen = RSA_ENCODE_LEN;
-
- //Get(&N, Modulus, 16);//string
- GetHex(&N, ModulusHex, RSA_ENCODE_LEN, 16);//hex array
- Get(&E, publicKey, 16);
-
- add_PKCS1Padding(data, len, buf);
-
- GetHex(&mw, buf, RSA_ENCODE_LEN, 16);
-
- RsaTrans(&mw, &E, &N);
-
- PutHex(&mw, out, &outlen);
- return outlen;
- }
-
- int RSA2048_pub_PKCS1Padding_Encode(unsigned char *data, unsigned short len, unsigned char *out, char *publicKey, unsigned char *ModulusHex)
- {
- unsigned char buf[RSA_ENCODE_LEN];
- CBigInt N, E;
- CBigInt mw, mi, jm;
- uint16_t outlen = RSA_ENCODE_LEN;
-
- GetHex(&N, ModulusHex, RSA_ENCODE_LEN, 16);//hex array
- Get(&E, publicKey, 16);
-
- add_PKCS1Padding(data, len, buf);
-
- GetHex(&mw, buf, RSA_ENCODE_LEN, 16);
-
- RsaTrans(&mw, &E, &N);
-
- PutHex(&mw, out, &outlen);
- return outlen;
- }
-
- int RSA2048_pri_PKCS1Padding_Decode(unsigned char *data, unsigned short *len, unsigned char *out, char *privateKey, char *ModulusHex)
- {
- unsigned char buf[RSA_ENCODE_LEN];
- CBigInt N, D;
- CBigInt mw, jm;
-
- //Get(&N, Modulus, 16);//string
- GetHex(&N, ModulusHex, RSA_ENCODE_LEN, 16);//hex array
- Get(&D, privateKey, 16);
-
- GetHex(&mw, data, *len, 16);
-
- RsaTrans(&mw, &D, &N);
- PutHex(&mw, buf, len);
- PKCS1PKCS1PaddingHexRemove(buf, len, out);
- return 0;
- }
- int RSA2048_pub_PKCS1Padding_Decode(unsigned char *data, unsigned short *len, unsigned char *out, char *privateKey, unsigned char *ModulusHex)
- {
- unsigned char buf[RSA_ENCODE_LEN];
- CBigInt N, D;
- CBigInt mw, jm;
- int t_len = 0;
-
- GetHex(&N, ModulusHex, RSA_ENCODE_LEN, 16);
- Get(&D, privateKey, 16);
-
- GetHex(&mw, data, *len, 16);
-
- RsaTrans(&mw, &D, &N);
- PutHex(&mw, buf, len);
- t_len = PKCS1PKCS1PaddingHexRemove(buf, len, out);
- return t_len;
- }
-
-
- //test
- static const unsigned char base64_table[65] = "ABCDEFGHIJKLMNOPQRSTUVWXYZabcdefghijklmnopqrstuvwxyz0123456789+/";
-
- //需要释放内存
- unsigned char * base64_encode(const unsigned char *src, size_t len, size_t *out_len)
- {
- unsigned char *out, *pos;
- const unsigned char *end, *in;
- size_t olen;
- int line_len;
-
- olen = len * 4 / 3 + 4; /* 3-byte blocks to 4-byte */
- olen += olen / 72; /* line feeds */
- olen++; /* nul termination */
- if(olen < len)
- {
- return NULL; /* integer overflow */
- }
- out = portable_malloc(olen);
- if(out == NULL)
- {
- return NULL;
- }
-
- end = src + len;
- in = src;
- pos = out;
- line_len = 0;
- while(end - in >= 3)
- {
- *pos++ = base64_table[in[0] >> 2];
- *pos++ = base64_table[((in[0] & 0x03) << 4) | (in[1] >> 4)];
- *pos++ = base64_table[((in[1] & 0x0f) << 2) | (in[2] >> 6)];
- *pos++ = base64_table[in[2] & 0x3f];
- in += 3;
- line_len += 4;
- if(line_len >= 72)
- {
- *pos++ = '\n';
- line_len = 0;
- }
- }
-
- if(end - in)
- {
- *pos++ = base64_table[in[0] >> 2];
- if(end - in == 1)
- {
- *pos++ = base64_table[(in[0] & 0x03) << 4];
- *pos++ = '=';
- }
- else
- {
- *pos++ = base64_table[((in[0] & 0x03) << 4) |
- (in[1] >> 4)];
- *pos++ = base64_table[(in[1] & 0x0f) << 2];
- }
- *pos++ = '=';
- line_len += 4;
- }
-
- if(line_len)
- {
- *pos++ = '\n';
- }
-
- *pos = '\0';
- if(out_len)
- {
- *out_len = pos - out;
- }
- return out;
- }
-
- //需要释放内存
- unsigned char * base64_decode(const unsigned char *src, size_t len,
- size_t *out_len)
- {
- unsigned char dtable[256], *out, *pos, block[4], tmp;
- size_t i, count, olen;
- int pad = 0;
-
- memset(dtable, 0x80, 256);
- for(i = 0; i < sizeof(base64_table) - 1; i++)
- {
- dtable[base64_table[i]] = (unsigned char) i;
- }
- dtable['='] = 0;
-
- count = 0;
- for(i = 0; i < len; i++)
- {
- if(dtable[src[i]] != 0x80)
- {
- count++;
- }
- }
-
- if(count == 0 || count % 4)
- {
- return NULL;
- }
-
- olen = count / 4 * 3;
- pos = out = portable_malloc(olen);
- if(out == NULL)
- {
- return NULL;
- }
-
- count = 0;
- for(i = 0; i < len; i++)
- {
- tmp = dtable[src[i]];
- if(tmp == 0x80)
- {
- continue;
- }
-
- if(src[i] == '=')
- {
- pad++;
- }
- block[count] = tmp;
- count++;
- if(count == 4)
- {
- *pos++ = (block[0] << 2) | (block[1] >> 4);
- *pos++ = (block[1] << 4) | (block[2] >> 2);
- *pos++ = (block[2] << 6) | block[3];
- count = 0;
- if(pad)
- {
- if(pad == 1)
- {
- pos--;
- }
- else if(pad == 2)
- {
- pos -= 2;
- }
- else
- {
- /* Invalid padding */
- portable_free(out);
- return NULL;
- }
- break;
- }
- }
- }
-
- *out_len = pos - out;
- return out;
- }
-
- int main(int argc, char *argv[])
- {
- //原始公钥-私钥
- /*
- -----BEGIN PUBLIC KEY-----
- MIIBIjANBgkqhkiG9w0BAQEFAAOCAQ8AMIIBCgKCAQEAwmejRhw/SB2xB3rgJYhg
- OWBDX/DponDVVPzTWhn3J4INv6jUa9HDkeHhys4OOZTNajr8kRy4TIemotnIYONJ
- noW7VyIQEAkEyxcMett5mqRPBLuyc8Fygn4ho/rd9JId4+PgKLmr6NVcuZCpVXPe
- gyqNx0nR/UojISbq/Bu+NlcStmicZUuAeVbkGGUOlvtMzFehkBPwE31EdpYUq+/z
- LuJ8OaxC+zm5PFo2AZJfI5Gz5lgb1g5ud0TG1JUrm9Dl5/JSNSL3SXBEC77mdfd0
- BA5VFl8lV7IfTfSTUE9IKoMevqZxoaGpyN+ZcBby5NgsoqJJ6vmcJRFjI92UrFHV
- 1wIDAQAB
- -----END PUBLIC KEY-----
-
- -----BEGIN PRIVATE KEY-----
- MIIEvQIBADANBgkqhkiG9w0BAQEFAASCBKcwggSjAgEAAoIBAQDCZ6NGHD9IHbEH
- euAliGA5YENf8OmicNVU/NNaGfcngg2/qNRr0cOR4eHKzg45lM1qOvyRHLhMh6ai
- 2chg40mehbtXIhAQCQTLFwx623mapE8Eu7JzwXKCfiGj+t30kh3j4+Aouavo1Vy5
- kKlVc96DKo3HSdH9SiMhJur8G742VxK2aJxlS4B5VuQYZQ6W+0zMV6GQE/ATfUR2
- lhSr7/Mu4nw5rEL7Obk8WjYBkl8jkbPmWBvWDm53RMbUlSub0OXn8lI1IvdJcEQL
- vuZ193QEDlUWXyVXsh9N9JNQT0gqgx6+pnGhoanI35lwFvLk2Cyioknq+ZwlEWMj
- 3ZSsUdXXAgMBAAECggEAEnEUaw0475VpesUsSEM0pZy9J3fKIg/EHQjS3+RAru3G
- ch0I8aV3gPpFmiCL9uhnyCEKXpWz4gaoRyCTwqUtEa2sBOsFTRAd9Uodc/YoBgR6
- Pn+zwQlj3H8sn8qnjZDi5wBx/ksGxNKgtjXD6ohQXm8F/hbBpd6HkJiJiBr1o1/a
- 4Kzh9L1OpYVS5QF0EN3idNkt911vfjA+bqBXXjAf9IHvirQ2GeCNzmHygxiRhAWA
- ki2fm0pGbKFUAfo+sgtDPT2jrA4rhSHG4mMr8IWBpr082aKNns7ujxT9ndBCl0Pp
- 7efPNCT4+zqfyVAB6x2rYhtXTqR7wsASMIfSLzyoIQKBgQDi2sb9z4OEl0mTV0Ey
- 1Lia4F06QhD7Kl1mhBWYPA1RO1ieAHHINFvwPJQLjHR2yMRMDmTOc3S9Hy3H3QMG
- XZlrIl5sgeFzsdqGyd2kscGFUGkH9RY2V/XB0mxQFIGI2PBztqd6e6BJQz4oSyMz
- cBZq/NH5u95QRlyI0I1u5b5lMQKBgQDbYZdhqHcDYmc61aHRNEhnxbK4ph1bdTiM
- QbIF0wUssXQKwEzvYzswRlI27rXeSHpJA8vc+XAoD/+Ut30I8Td3yUnnsHZctU2D
- b/8OTqwFqyrwXM+SvyWZejuvX6IVJuRwPMFG88L88lryEs0ntlQKC/x6wxtcs67X
- 6ZGsLSfJhwKBgQDAo09/kIv6OA4+lEXFSGZK/mOsaRXKcztFJry/vZ8BcAfchDwa
- 6nt4EbkV5XuwsuQeQcrQlbJ4NtXFdqRu72SsWU8djV1Jxanv89PHWzseXh4Sp8jo
- 9OC4aluX1RH6h14IpP6rP/fovrU1ujh2IaSnzXDxRNuQB2/krlSr62Q2wQKBgGNL
- jbgvBwcqH+06So6lKmyFx/nZfgoqSVj6VzhZpcrv2sUO+wOTF3QnMAkbDIg6p9aq
- eDhhUklfzF+kmVxVybRXEDNk5H3bteTa6Uexhhzet4WpjG4wRDVuZNtg3rzSKK1A
- Yn7Z0BSrIUzWA7OIzArsF+/8pULVNTsWxc93dL27AoGAT3eaPv07rhzyztxFYTsr
- dHcgNb+vwroN3Ic7IJnxoa2BjyqczaAG3vMrOcxFg2MLJ7fPmI1W4pPOJyxmUhO0
- mZhk+F7h+dg7XB7h0lqR1usp3Ak3qyu1f4XZj4imzQWWyETpgE4uA9tBniPQIRK6
- miXhZ2YoXfdsSZOatWojGLI=
- -----END PRIVATE KEY-----
- */
-
- //https://www.oren.net.cn/rsa/info.html
- //在线转换为模数和指数
-
- //PEM->模数+指数
- char *publicKey_exponent = "10001";
- char *privateKey_exponent = "1271146b0d38ef95697ac52c484334a59cbd2777ca220fc41d08d2dfe440aeedc6721d08f1a57780fa459a208bf6e867c8210a5e95b3e206a8472093c2a52d11adac04eb054d101df54a1d73f62806047a3e7fb3c10963dc7f2c9fcaa78d90e2e70071fe4b06c4d2a0b635c3ea88505e6f05fe16c1a5de87909889881af5a35fdae0ace1f4bd4ea58552e5017410dde274d92df75d6f7e303e6ea0575e301ff481ef8ab43619e08dce61f2831891840580922d9f9b4a466ca15401fa3eb20b433d3da3ac0e2b8521c6e2632bf08581a6bd3cd9a28d9eceee8f14fd9dd0429743e9ede7cf3424f8fb3a9fc95001eb1dab621b574ea47bc2c0123087d22f3ca821";
-
- //hex array ,faster than string
- //直接使用16进制数据可略微提高速度,软件也支持传入字符串
- // 具体看代码中 GetHex 和 Get
- static unsigned char ModulusHex[RSA_ENCODE_LEN] =
- {
- 0xC2, 0x67, 0xA3, 0x46, 0x1C, 0x3F, 0x48, 0x1D, 0xB1, 0x07, 0x7A, 0xE0, 0x25, 0x88, 0x60, 0x39,
- 0x60, 0x43, 0x5F, 0xF0, 0xE9, 0xA2, 0x70, 0xD5, 0x54, 0xFC, 0xD3, 0x5A, 0x19, 0xF7, 0x27, 0x82,
- 0x0D, 0xBF, 0xA8, 0xD4, 0x6B, 0xD1, 0xC3, 0x91, 0xE1, 0xE1, 0xCA, 0xCE, 0x0E, 0x39, 0x94, 0xCD,
- 0x6A, 0x3A, 0xFC, 0x91, 0x1C, 0xB8, 0x4C, 0x87, 0xA6, 0xA2, 0xD9, 0xC8, 0x60, 0xE3, 0x49, 0x9E,
- 0x85, 0xBB, 0x57, 0x22, 0x10, 0x10, 0x09, 0x04, 0xCB, 0x17, 0x0C, 0x7A, 0xDB, 0x79, 0x9A, 0xA4,
- 0x4F, 0x04, 0xBB, 0xB2, 0x73, 0xC1, 0x72, 0x82, 0x7E, 0x21, 0xA3, 0xFA, 0xDD, 0xF4, 0x92, 0x1D,
- 0xE3, 0xE3, 0xE0, 0x28, 0xB9, 0xAB, 0xE8, 0xD5, 0x5C, 0xB9, 0x90, 0xA9, 0x55, 0x73, 0xDE, 0x83,
- 0x2A, 0x8D, 0xC7, 0x49, 0xD1, 0xFD, 0x4A, 0x23, 0x21, 0x26, 0xEA, 0xFC, 0x1B, 0xBE, 0x36, 0x57,
- 0x12, 0xB6, 0x68, 0x9C, 0x65, 0x4B, 0x80, 0x79, 0x56, 0xE4, 0x18, 0x65, 0x0E, 0x96, 0xFB, 0x4C,
- 0xCC, 0x57, 0xA1, 0x90, 0x13, 0xF0, 0x13, 0x7D, 0x44, 0x76, 0x96, 0x14, 0xAB, 0xEF, 0xF3, 0x2E,
- 0xE2, 0x7C, 0x39, 0xAC, 0x42, 0xFB, 0x39, 0xB9, 0x3C, 0x5A, 0x36, 0x01, 0x92, 0x5F, 0x23, 0x91,
- 0xB3, 0xE6, 0x58, 0x1B, 0xD6, 0x0E, 0x6E, 0x77, 0x44, 0xC6, 0xD4, 0x95, 0x2B, 0x9B, 0xD0, 0xE5,
- 0xE7, 0xF2, 0x52, 0x35, 0x22, 0xF7, 0x49, 0x70, 0x44, 0x0B, 0xBE, 0xE6, 0x75, 0xF7, 0x74, 0x04,
- 0x0E, 0x55, 0x16, 0x5F, 0x25, 0x57, 0xB2, 0x1F, 0x4D, 0xF4, 0x93, 0x50, 0x4F, 0x48, 0x2A, 0x83,
- 0x1E, 0xBE, 0xA6, 0x71, 0xA1, 0xA1, 0xA9, 0xC8, 0xDF, 0x99, 0x70, 0x16, 0xF2, 0xE4, 0xD8, 0x2C,
- 0xA2, 0xA2, 0x49, 0xEA, 0xF9, 0x9C, 0x25, 0x11, 0x63, 0x23, 0xDD, 0x94, 0xAC, 0x51, 0xD5, 0xD7
- };
-
- unsigned char encode_str[RSA_ENCODE_LEN] = {0};
- unsigned char decode_str[RSA_ENCODE_LEN] = {0};
-
- int outlen = 0;
-
- unsigned char * base64_str = NULL;
- unsigned int base64_str_len = NULL;
-
- uint8_t* decode_base64 = NULL;
- char pub_source_string[] = "embedded-system > Public key to RSA encode,hehe";
- char pri_source_string[] = "embedded-system > Private key to RSA encode,haha";
-
- printf("RSA demo");
- //---------------------------------------------------
- memset(encode_str, 0, sizeof(encode_str));
- memset(decode_str, 0, sizeof(decode_str));
- //公钥加密
- outlen = RSA2048_pub_PKCS1Padding_Encode(pub_source_string, strlen(pub_source_string), encode_str, publicKey_exponent, ModulusHex);
-
- //密文转base64方便显示,和在线工具对比结果
- // https://the-x.cn/cryptography/Rsa.aspx
- //base64_str = base64_encode(encode_str, outlen, &base64_str_len);
- //printf("Public encode %d\r\n%s\r\n", base64_str_len, base64_str);
- //portable_free(base64_str);
-
- //私钥解密
- RSA2048_pri_PKCS1Padding_Decode(encode_str, &outlen, decode_str, privateKey_exponent, ModulusHex);
- printf("Private decode %d\r\n%s\r\n", outlen, decode_str);
-
- //---------------------------------------------------
- memset(encode_str, 0, sizeof(encode_str));
- memset(decode_str, 0, sizeof(decode_str));
-
- //私钥签名
- outlen = RSA2048_pri_PKCS1Padding_Encode(pri_source_string, strlen(pri_source_string), encode_str, privateKey_exponent, ModulusHex);
-
- //公钥验签
- RSA2048_pub_PKCS1Padding_Decode(encode_str, &outlen, decode_str, publicKey_exponent, ModulusHex);
- printf("Public decode %d\r\n%s\r\n", outlen, decode_str);
-
- //---------------------------------------------------
- printf("RSA demo done");
- return 0;
- }
-
-
和AES一样,RSA也是块加密算法( block cipher algorithm),只针对固定长度明文,如RSA2048其加密的明文长度需要填充后是2048位即256字节,如果明文长度大于256字节则需要拆分。当然最简单的办法是应用层分配256字节缓存,有效数据以外以0x00填充。
RSA算法虽然安全,但计算量非常大,效率较低,尤其在嵌入式系统中,硬件资源有限的情况下加密、解密时间以秒为单位。而对称加密算法AES算法效率高,但其在密钥协商时,在网络传输中有被拦截的风险,或者任一方保存不当导致密钥泄露,其密钥存在很大的安全隐患。
所以,考虑到安全性和高效性,一般采用多种算法组合加密的方式。使用RSA来加密AES的密钥,密钥协商后,使用AES来对后续数据进行加密。

